Integral.docx

Integral.docx

《Integral.docx》由会员分享，可在线阅读，更多相关《Integral.docx（38页珍藏版）》请在冰豆网上搜索。

Integral

Integral

FromWikipedia,thefreeencyclopedia

Thisarticleisabouttheconceptofdefiniteintegralsincalculus.Fortheindefiniteintegral,seeantiderivative.Forthesetofnumbers,seeinteger.Forotheruses,seeIntegral（disambiguation）.

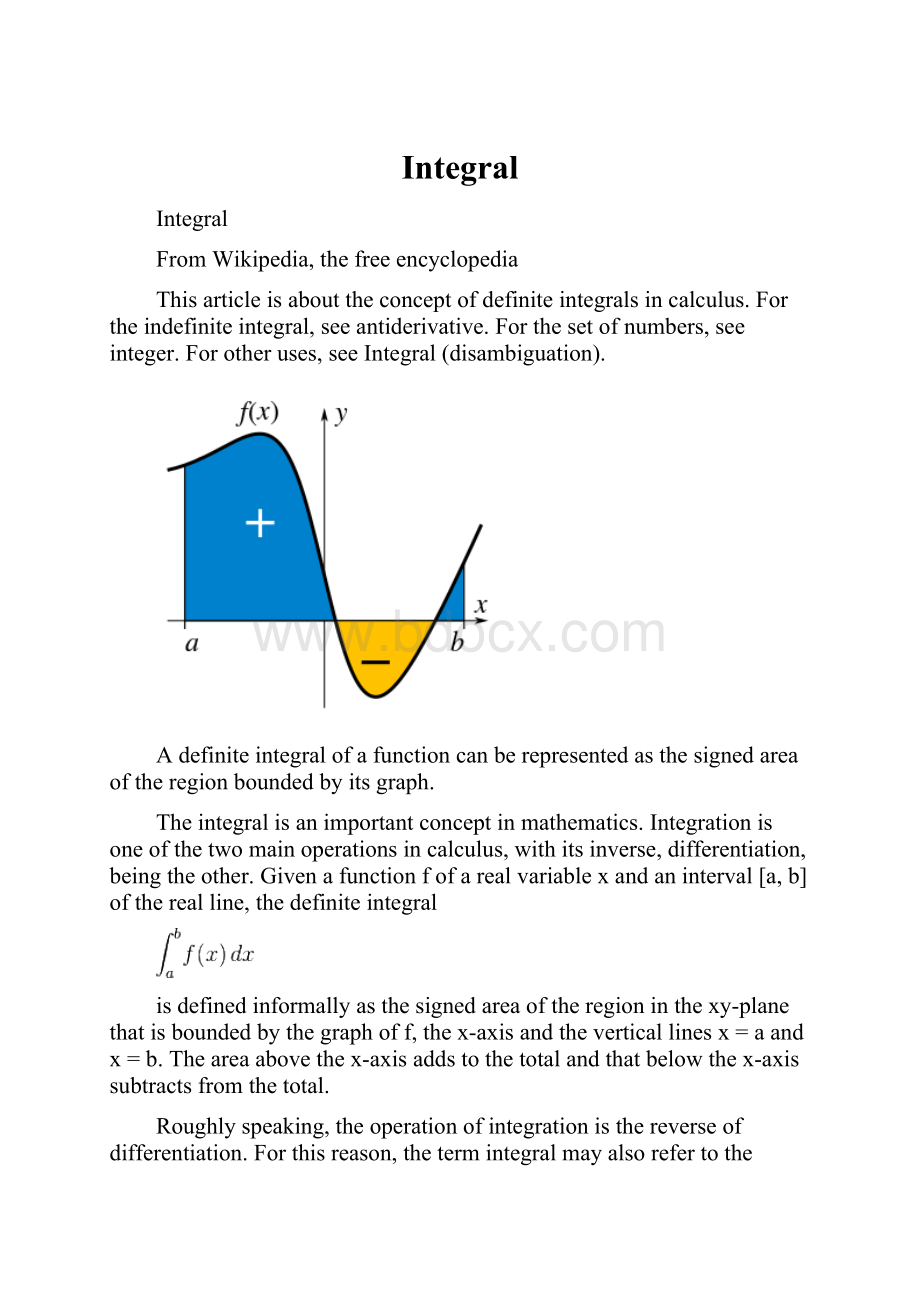
Adefiniteintegralofafunctioncanberepresentedasthesignedareaoftheregionboundedbyitsgraph.

Theintegralisanimportantconceptinmathematics.Integrationisoneofthetwomainoperationsincalculus,withitsinverse,differentiation,beingtheother.Givenafunctionfofarealvariablexandaninterval[a,b]oftherealline,thedefiniteintegral

isdefinedinformallyasthesignedareaoftheregioninthexy-planethatisboundedbythegraphoff,thex-axisandtheverticallinesx=aandx=b.Theareaabovethex-axisaddstothetotalandthatbelowthex-axissubtractsfromthetotal.

Roughlyspeaking,theoperationofintegrationisthereverseofdifferentiation.Forthisreason,thetermintegralmayalsorefertotherelatednotionoftheantiderivative,afunctionFwhosederivativeisthegivenfunctionf.Inthiscase,itiscalledanindefiniteintegralandiswritten:

Theintegralsdiscussedinthisarticlearethosetermeddefiniteintegrals.Itisthefundamentaltheoremofcalculusthatconnectsdifferentiationwiththedefiniteintegral:

iffisacontinuousreal-valuedfunctiondefinedonaclosedinterval[a,b],then,onceanantiderivativeFoffisknown,thedefiniteintegraloffoverthatintervalisgivenby

TheprinciplesofintegrationwereformulatedindependentlybyIsaacNewtonandGottfriedLeibnizinthelate17thcentury,whothoughtoftheintegralasaninfinitesumofrectanglesofinfinitesimalwidth.ArigorousmathematicaldefinitionoftheintegralwasgivenbyBernhardRiemann.Itisbasedonalimitingprocedurewhichapproximatestheareaofacurvilinearregionbybreakingtheregionintothinverticalslabs.Beginninginthenineteenthcentury,moresophisticatednotionsofintegralsbegantoappear,wherethetypeofthefunctionaswellasthedomainoverwhichtheintegrationisperformedhasbeengeneralised.Alineintegralisdefinedforfunctionsoftwoorthreevariables,andtheintervalofintegration[a,b]isreplacedbyacertaincurveconnectingtwopointsontheplaneorinthespace.Inasurfaceintegral,thecurveisreplacedbyapieceofasurfaceinthethree-dimensionalspace.

Integralsofdifferentialformsplayafundamentalroleinmoderndifferentialgeometry.Thesegeneralizationsofintegralsfirstarosefromtheneedsofphysics,andtheyplayanimportantroleintheformulationofmanyphysicallaws,notablythoseofelectrodynamics.Therearemanymodernconceptsofintegration,amongthese,themostcommonisbasedontheabstractmathematicaltheoryknownasLebesgueintegration,developedbyHenriLebesgue.

Contents

∙1History

o1.1Pre-calculusintegration

o1.2NewtonandLeibniz

o1.3Formalization

o1.4Historicalnotation

∙2Terminologyandnotation

∙3Interpretationsoftheintegral

∙4Formaldefinitions

o4.1Riemannintegral

o4.2Lebesgueintegral

o4.3Otherintegrals

∙5Properties

o5.1Linearity

o5.2Inequalities

o5.3Conventions

∙6Fundamentaltheoremofcalculus

o6.1Statementsoftheorems

▪6.1.1Fundamentaltheoremofcalculus

▪6.1.2Secondfundamentaltheoremofcalculus

∙7Extensions

o7.1Improperintegrals

o7.2Multipleintegration

o7.3Lineintegrals

o7.4Surfaceintegrals

o7.5Integralsofdifferentialforms

o7.6Summations

∙8Computation

o8.1Analytical

o8.2Symbolic

o8.3Numerical

o8.4Mechanical

o8.5Geometrical

∙9Someimportantdefiniteintegrals

∙10Seealso

∙11Notes

∙12References

∙13Externallinks

o13.1Onlinebooks

History

Seealso:

Historyofcalculus

Pre-calculusintegration

ThefirstdocumentedsystematictechniquecapableofdeterminingintegralsisthemethodofexhaustionoftheancientGreekastronomerEudoxus（ca.370BC）,whichsoughttofindareasandvolumesbybreakingthemupintoaninfinitenumberofdivisionsforwhichtheareaorvolumewasknown.ThismethodwasfurtherdevelopedandemployedbyArchimedesinthe3rdcenturyBCandusedtocalculateareasforparabolasandanapproximationtotheareaofacircle.SimilarmethodswereindependentlydevelopedinChinaaroundthe3rdcenturyADbyLiuHui,whousedittofindtheareaofthecircle.Thismethodwaslaterusedinthe5thcenturybyChinesefather-and-sonmathematiciansZuChongzhiandZuGengtofindthevolumeofasphere（Shea2007;Katz2004,pp. 125–126）.

Thenextsignificantadvancesinintegralcalculusdidnotbegintoappearuntilthe16thcentury.AtthistimetheworkofCavalieriwithhismethodofIndivisibles,andworkbyFermat,begantolaythefoundationsofmoderncalculus,withCavaliericomputingtheintegralsofxnuptodegreen=9inCavalieri'squadratureformula.Furtherstepsweremadeintheearly17thcenturybyBarrowandTorricelli,whoprovidedthefirsthintsofaconnectionbetweenintegrationanddifferentiation.Barrowprovidedthefirstproofofthefundamentaltheoremofcalculus.WallisgeneralizedCavalieri'smethod,computingintegralsofxtoageneralpower,includingnegativepowersandfractionalpowers.

NewtonandLeibniz

Themajoradvanceinintegrationcameinthe17thcenturywiththeindependentdiscoveryofthefundamentaltheoremofcalculusbyNewtonandLeibniz.Thetheoremdemonstratesaconnectionbetweenintegrationanddifferentiation.Thisconnection,combinedwiththecomparativeeaseofdifferentiation,canbeexploitedtocalculateintegrals.Inparticular,thefundamentaltheoremofcalculusallowsonetosolveamuchbroaderclassofproblems.EqualinimportanceisthecomprehensivemathematicalframeworkthatbothNewtonandLeibnizdeveloped.Giventhenameinfinitesimalcalculus,itallowedforpreciseanalysisoffunctionswithincontinuousdomains.Thisframeworkeventuallybecamemoderncalculus,whosenotationforintegralsisdrawndirectlyfromtheworkofLeibniz.

Formalization

WhileNewtonandLeibnizprovidedasystematicapproachtointegration,theirworklackedadegreeofrigour.BishopBerkeleymemorablyattackedthevanishingincrementsusedbyNewton,callingthem"ghostsofdepartedquantities".Calculusacquiredafirmerfootingwiththedevelopmentoflimits.Integrationwasfirstrigorouslyformalized,usinglimits,byRiemann.AlthoughallboundedpiecewisecontinuousfunctionsareRiemannintegrableonaboundedinterval,subsequentlymoregeneralfunctionswereconsidered—particularlyinthecontextofFourieranalysis—towhichRiemann'sdefinitiondoesnotapply,andLebesgueformulatedadifferentdefinitionofintegral,foundedinmeasuretheory（asubfieldofrealanalysis）.Otherdefinitionsofintegral,extendingRiemann'sandLebesgue'sapproaches,wereproposed.Theseapproachesbasedontherealnumbersystemaretheonesmostcommontoday,butalternativeapproachesexist,suchasadefinitionofintegralasthestandardpartofaninfiniteRiemannsum,basedonthehyperrealnumbersystem.

Historicalnotation

IsaacNewtonusedasmallverticalbaraboveavariabletoindicateintegration,orplacedthevariableinsideabox.Theverticalbarwaseasilyconfusedwith.xorx′,whichNewtonusedtoindicatedifferentiation,andtheboxnotationwasdifficultforprinterstoreproduce,sothesenotationswerenotwidelyadopted.

ThemodernnotationfortheindefiniteintegralwasintroducedbyGottfriedLeibnizin1675（Burton1988,p. 359;Leibniz1899,p. 154）.Headaptedtheintegralsymbol,∫,fromtheletterſ（longs）,standingforsumma（writtenasſumma;Latinfor"sum"or"total"）.Themodernnotationforthedefiniteintegral,withlimitsaboveandbelowtheintegralsign,wasfirstusedbyJosephFourierinMémoiresoftheFrenchAcademyaround1819–20,reprintedinhisbookof1822（Cajori1929,pp. 249–250;Fourier1822,§231）.

Terminologyandnotation

Thesimplestcase,theintegralwithrespecttoxofareal-valuedfunctionf（x）,iswrittenas

Theintegralsign∫representsintegration.Thesymboldx（explainedbelow）indicatesthevariableofintegration,x.Thefunctionf（x）whichistobeintegratediscalledtheintegrand.Incorrectmathematicaltypography,thedxisseparatedfromtheintegrandbyaspace（asshown）.Someauthorsuseanuprightd（thatis,dxinsteadofdx）.Also,someauthorsplacethesymboldxbeforef（x）ratherthanafterit.Becausethereisnodomainspecified,theaboveintegraliscalledanindefiniteintegral.

Whenintegratingoveraspecifieddomain,wespeakofadefiniteintegral.IntegratingoveradomainDiswrittenas

.IfDisaninterval[a,b]oftherealline,theintegralisusuallywritten

.ThedomainDortheinterval[a,b]iscalledthedomainofintegration.

Ifafunctionhasanintegral,itissaidtobeintegrable.Ingeneral,theintegrandmaybeafunctionofmorethanonevariable,andthedomainofintegrationmaybeanarea,volume,ahigher-dimensionalregion,orevenanabstractspacethatdoesnothaveageometricstructureinanyusualsense（suchasasamplespaceinprobabilitytheory）.

InmodernArabicmathematicalnotation,areflectedintegralsymbol

isused（W3C2006）.

Thesymboldxhasdifferentinterpretationsdependingonthetheorybeingused.InLeibniz'snotation,dxisinterpretedasaninfinitesimalchangeinx.AlthoughLeibniz'sinterpretationlacksrigour,hisintegrationnotationisthemostcommononeinusetoday.Iftheunderlyingtheoryofintegrationisnotimportant,dxcanbeseenasstrictlyanotationindicatingthatxisadummyvariableofintegration;iftheintegralisseenasaRiemannintegral,dxindicatesth